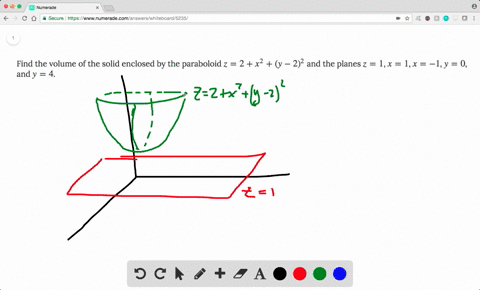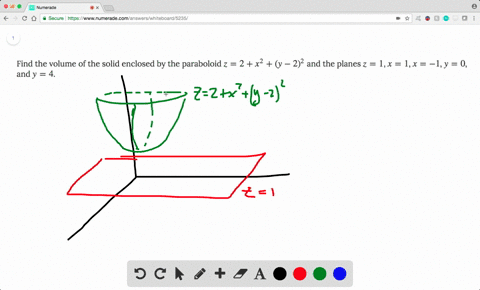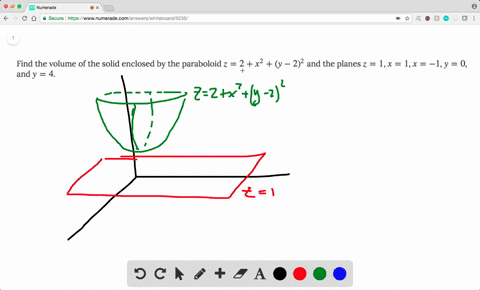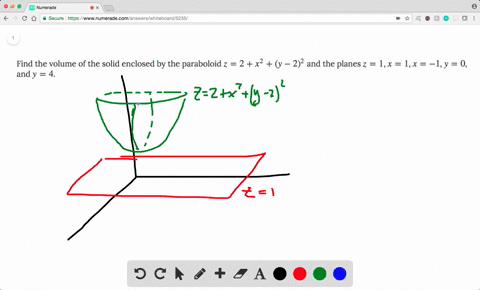
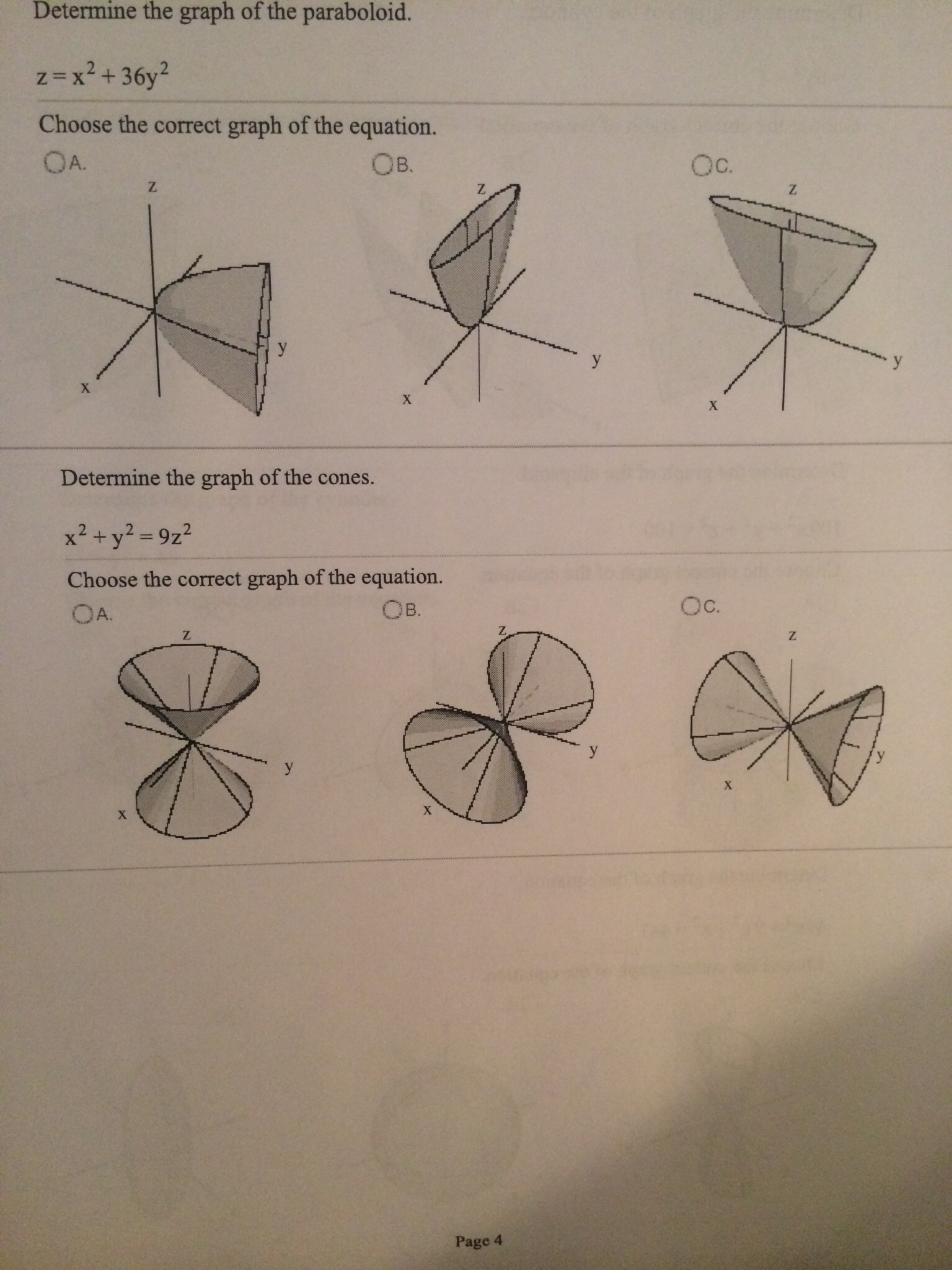
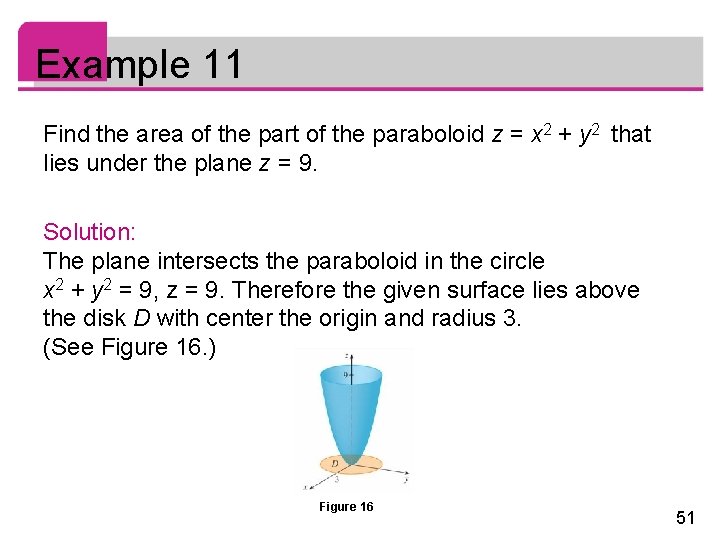
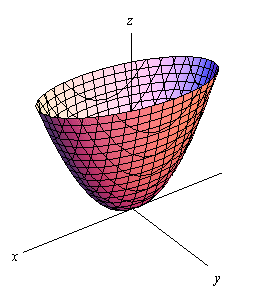
Quadricsurfs Html
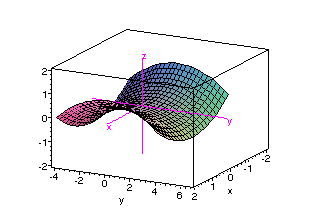
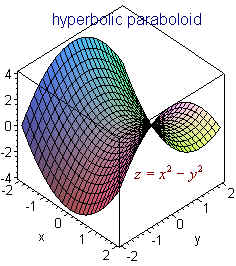
Experts are tested by Chegg as specialists in their subject area We review their content and use your feedback to keep the quality high 100% (1 rating)When z = x 2 y 2, the trace on y = b is the graph of z = x 2 b 2, while that on x = a is the graph of z = a 2 y 2;
Graph of paraboloid z=x^2 y^2
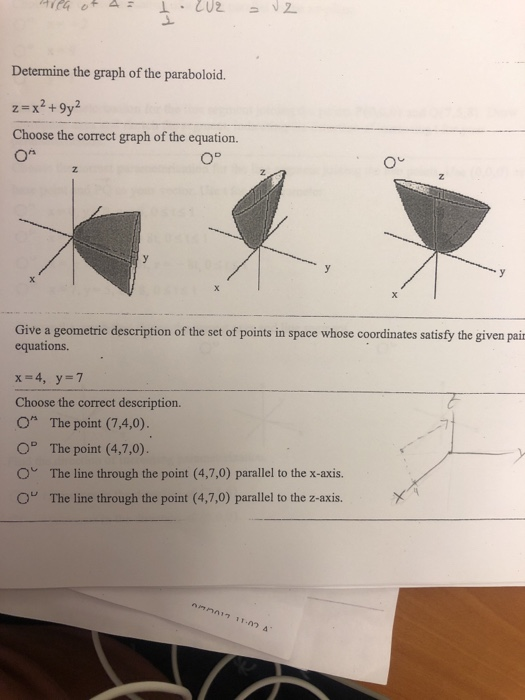
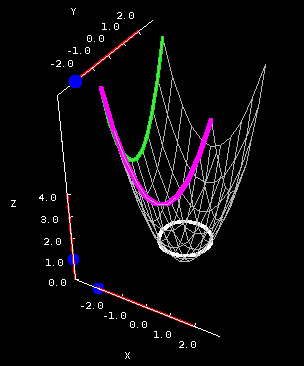
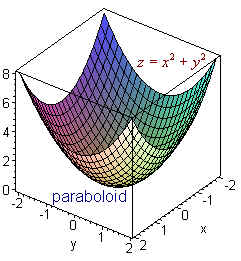
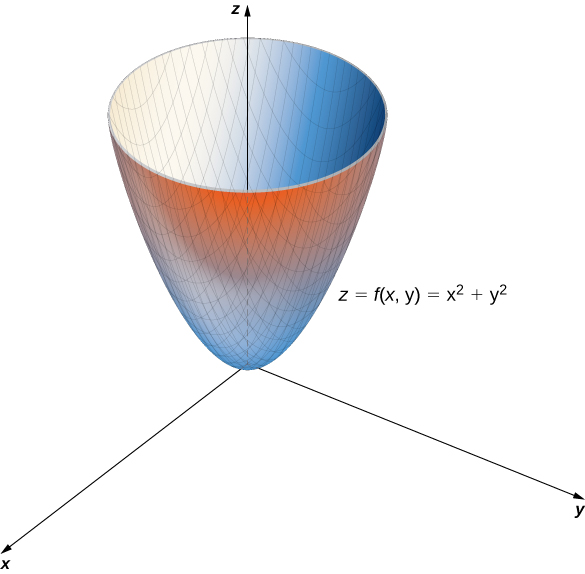
Graph of paraboloid z=x^2 y^2-Answer Okay, so we have z = x^2 y^2 describing the paraboloid and we have x^2 y^2 = 2y describing the cylinder That's how they look like together We want the equation describing the cylinder to be in its conventional form for simplicity We can subtract the The three dimensional graph with axes labeled x, y, and z, is of a concave upward circular paraboloid z = x 2 y 2, is centered at the origin 0 0 0The bottom part of the paraboloid is tangent to the origin and is labeled as a local minimum Any horizontal slice of the paraboloid will result in a circle
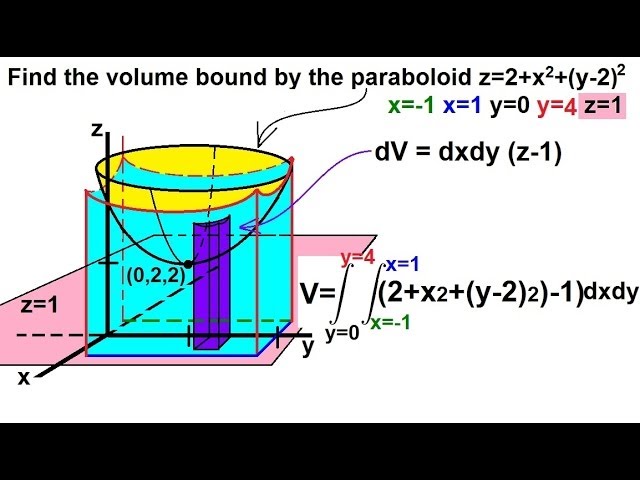
What Is The Volume Between Paraboloid Z X 2 Y 2 And Y X 2 Z 2 Quora
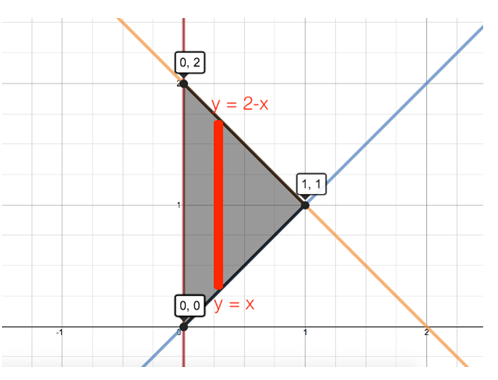
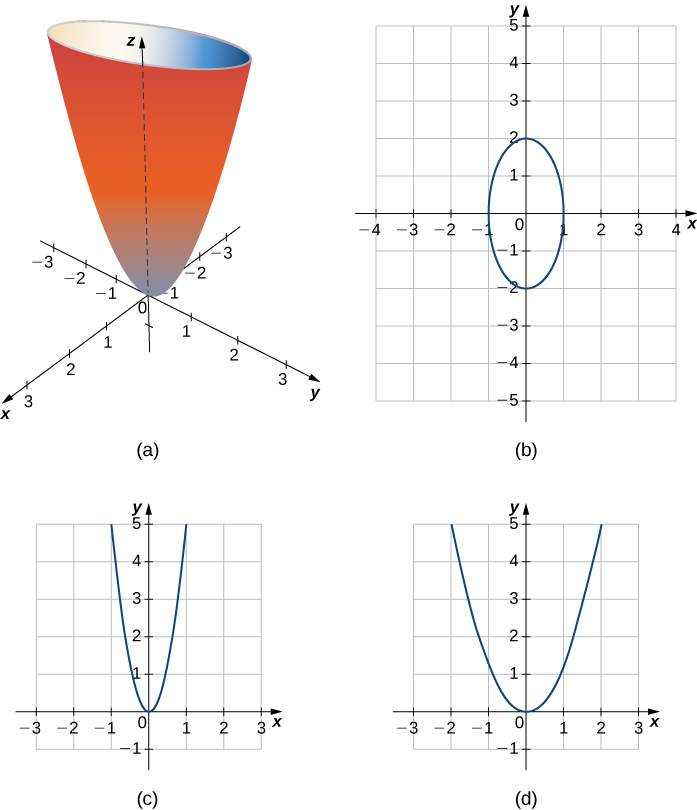
In the twodimensional coordinate plane, the equation x 2 y 2 = 9 x 2 y 2 = 9 describes a circle centered at the origin with radius 3 3 In threedimensional space, this same equation represents a surface Imagine copies of a circle stacked on top of each other centered on the zaxis (Figure 275), forming a hollow tube Z=x^2y^2 WolframAlpha Volume of a cylinder?(e) Below is the graph of z = x2 y2 On the graph of the surface, sketch the traces that you found in parts (a) and (c) For problems 1213, nd an equation of the trace of the surface in the indicated plane Describe the graph of the trace 12 Surface 8x 2 y z2 = 9;It's the equation of sphere The general equation of sphere looks likeGraph of paraboloid z=1x^2y^2Please ask as separate question(s) if any of these are not already established Concept of partial derivatives The area of a surface, f(x,y), above a region R of the XYplane is given by int int_R sqrt((f_x')^2 (f_y')^2 1) dx dy where f_x' and f_y' are the partial derivatives of f(x,y) with respect to x and y respectively In converting the integral of aZ = x2 y2
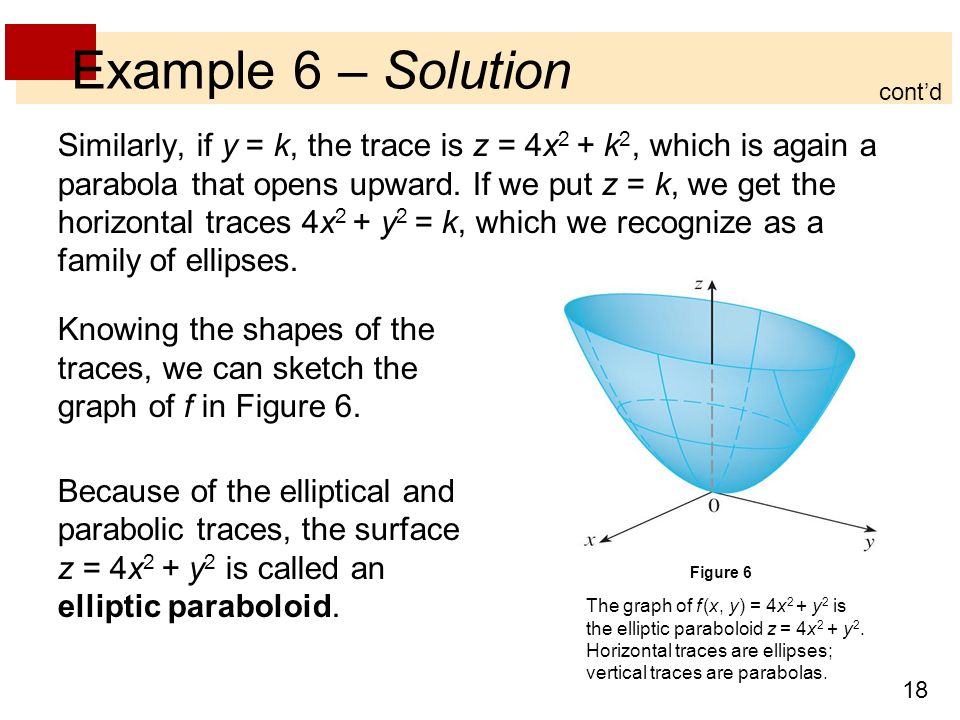
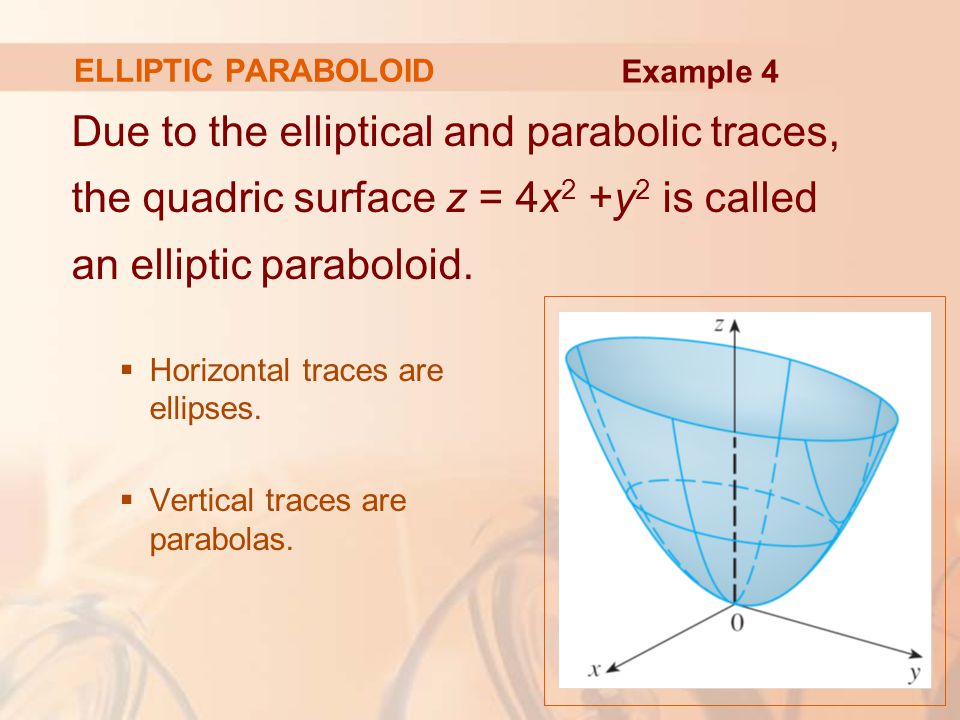
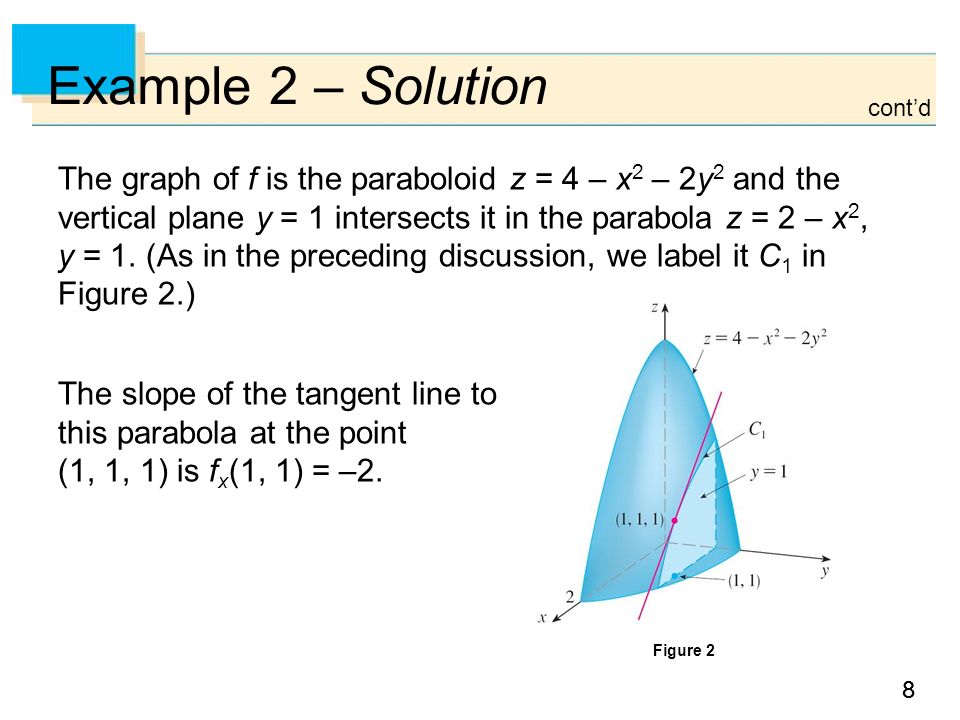
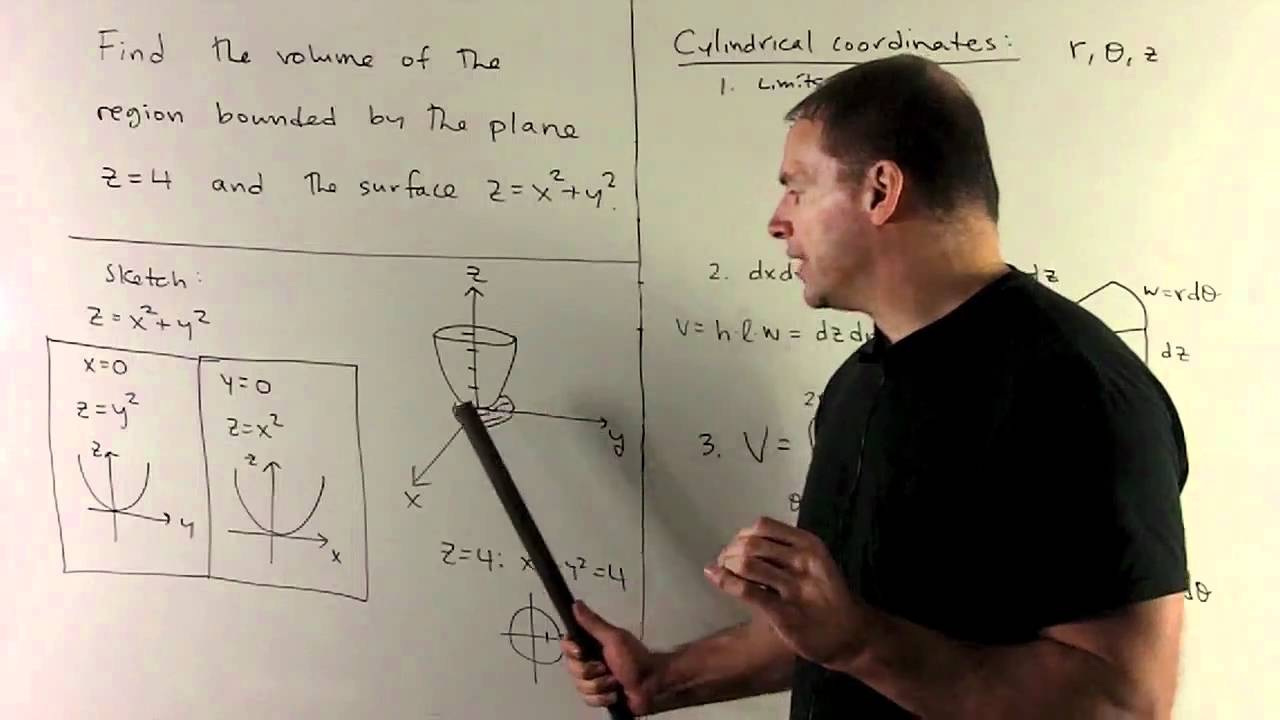
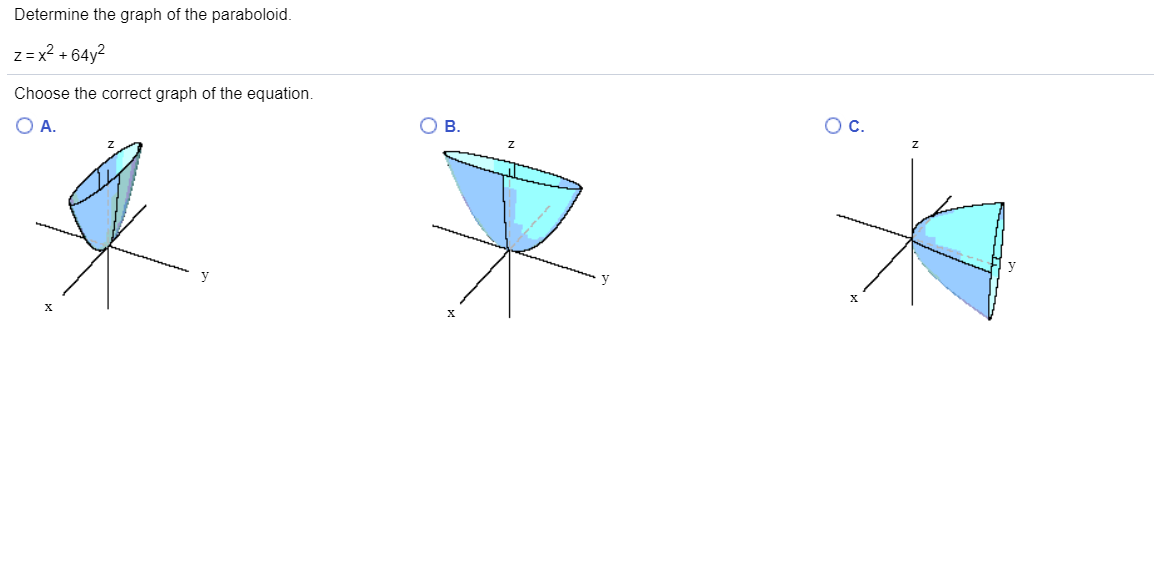
I originally read your "zx" as z x, but now that I see you mean z = y^2 / x, it makes a lot more sense Indeed, there are asymptotes going on here The above graph looks like this There is a vertical "wall" that should not actually appear in the graph it is actually the asymptote Here I've added z = x^2 / y Next, here are all 4 of yourExample 2 A circular paraboloid Start with a pair of zx axes and draw the parabola =16− 2 Now introduce a new horizontal yaxis so that we have a 3D coordinate system Rotate the parabola about the zaxis through 360° What we get is called aI assume the following knowledge;Answer to Find the area of the paraboloid z = 1 x^2 y^2 that lies in the first octant By signing up, you'll get thousands of stepbystepAmong all the points on the graph of z=9−x^2−y^2 that lie above the plane x2y6z= 0, find the point farthest from the planeGraph y=9x^2 Find the properties of the given parabola Tap for more steps Rewrite the
Graph of paraboloid z=x^2 y^2のギャラリー
各画像をクリックすると、ダウンロードまたは拡大表示できます
 |  | |
 |  | |
 |  | |
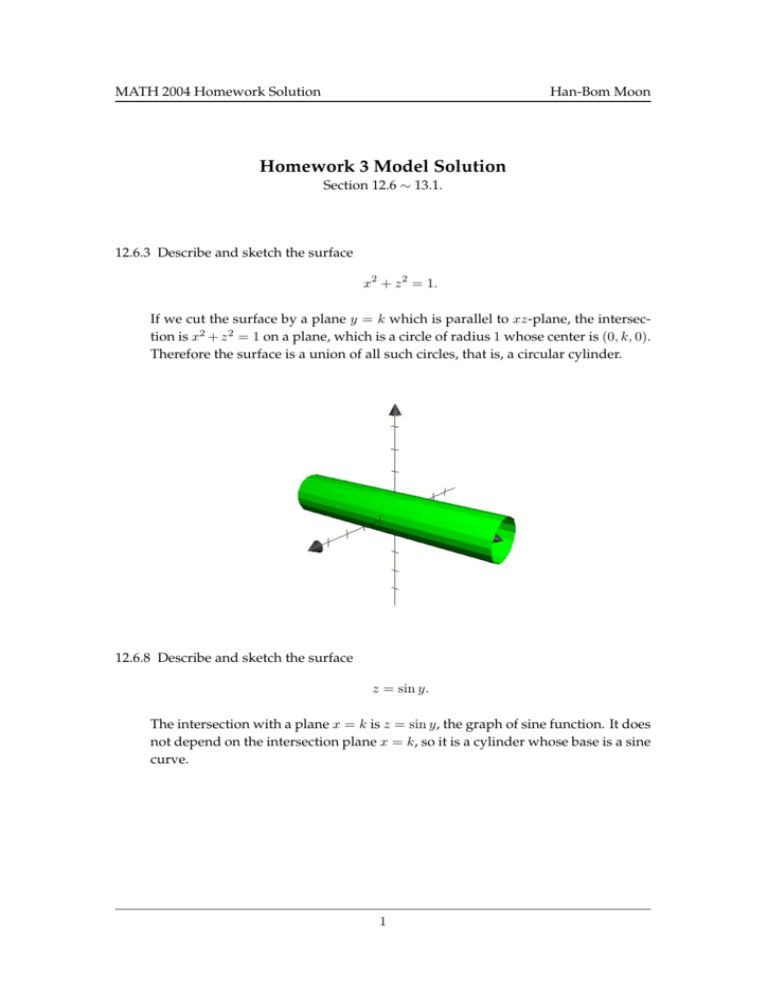
「Graph of paraboloid z=x^2 y^2」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  | |
 |  |  |
 | ||
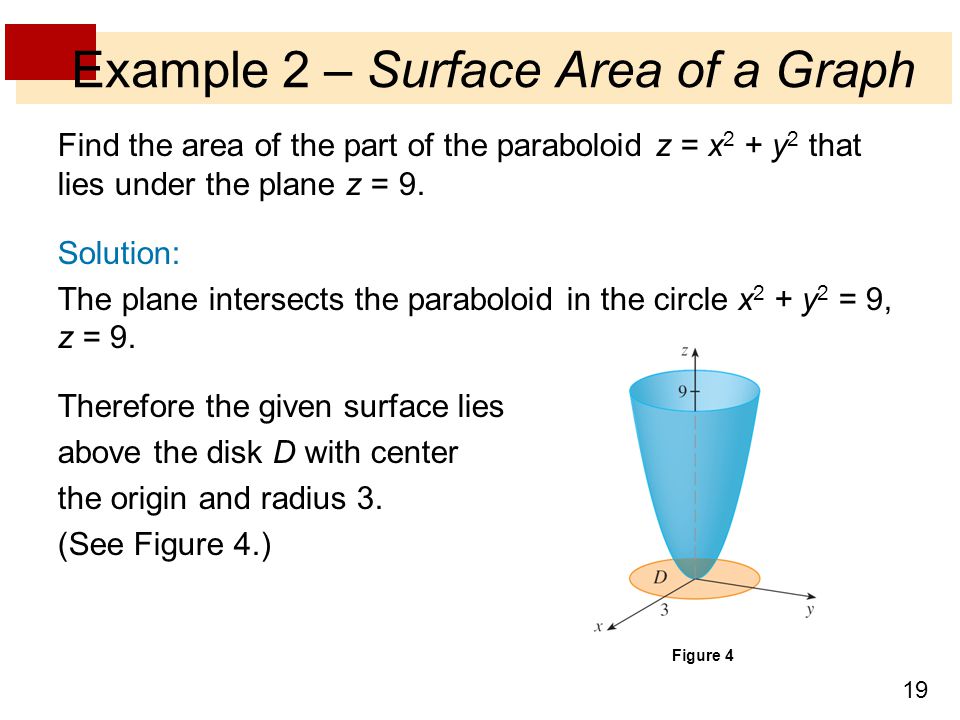
「Graph of paraboloid z=x^2 y^2」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  |  |
 | ||
 |  | |
「Graph of paraboloid z=x^2 y^2」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  | |
 |  |  |
 |  |  |
「Graph of paraboloid z=x^2 y^2」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  | |
 |  | |
 |  | |
「Graph of paraboloid z=x^2 y^2」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  | |
 |  | |
 |  | |
「Graph of paraboloid z=x^2 y^2」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  | |
 | ||
 |  | |
「Graph of paraboloid z=x^2 y^2」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  | |
 | 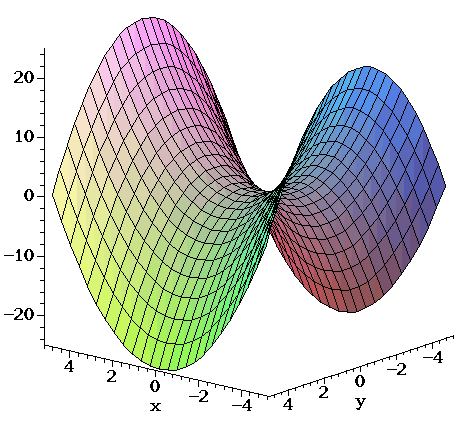 | |
 |  | |
「Graph of paraboloid z=x^2 y^2」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  |  |
 |  | |
 |  | |
「Graph of paraboloid z=x^2 y^2」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  | |
 |  | |
 |  |  |
「Graph of paraboloid z=x^2 y^2」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  | |
 |  |  |
 |  | |
「Graph of paraboloid z=x^2 y^2」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  | |
 |  |
As long as you have the mathematical equation describing that hyperboloid, you should be able to generate some data and then draw it Take a unit sphere for example, the equation is x^2y^2z^2=1;Z=x^2 y^2 graph Graph of paraboloid z=x^2y^2 Surfaces and Contour Plots Part 4 Graphs of Functions of Two Variables The graph of a function z = f(x,y) is also the graph of an equation in three variables and is therefore a surfaceSince each pair (x,y)




